A couple new article relevant to our study of Earth History.
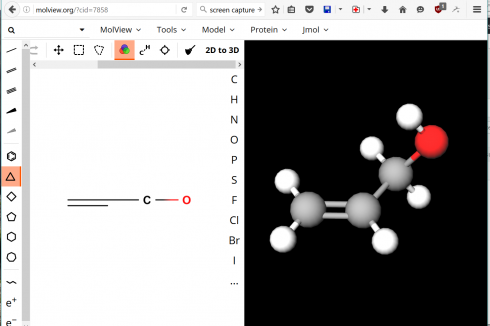
Carbon
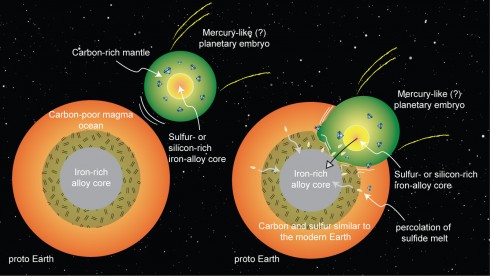
Research on the high pressure and temperature conditions at the Earth’s core suggest that most of the carbon in the early Earth should have either boiled off into space or been trapped by the iron in the core. So where did all the carbon necessary for life come from? They suggest from the collision of an embryonic planet (with lots of carbon in its upper layers) early in the formation of the solar system.
Free Oxygen
It took a few billion years from the evolution of the first photosynthetic cyanobacteria to the time when there was enough oxygen in the atmosphere to support animal life like us. Why did it take so long? NPR interviews scientists investigating purple microbial mats in Lake Huron.