The Draining of a Plastic Bottle: Integrating a Physics Experiment into Calculus

Abstract

I punched a small hole (about 1mm radius) in a one gallon plastic bottle and had my students measure the rate at which water drained. Even though the apparatus and measurement technique was fairly rough, we were able to, with a little calculus, determine the equation for the height of the water in the bottle as a function of time.

calculus_jug_1110 — Figure 1. A student uses a stopwatch to measure the outflow rate of water from the plastic bottle.

Introduction

Questions about water draining from a tank are a pretty common in calculus textbooks, but there is a significant difference between seeing the problem written down, and having to figure it out from a physical example. The latter is much more challenging because it does not presuppose any relationships for the change in the height of water with time; students must determine the relationship from the data they collect.

The experimental approach mimics the challenges faced by scientists such as Henry Darcy who first determined the formulas for groundwater flow (Darcy, 1733) almost 300 years ago, not long after the development of modern calculus by Newton and Leibniz (O’Conner and Robertson, 1996).

Procedure

I punched a small hole, about 1mm in radius, in the base of a plastic, one-gallon bottle. I chose this particular type of bottle because the bulk of it was cylindrical in shape.

Students were instructed to figure out how the rate at which water flowed out (outflow rate) changed with time, and how the height of the water (h) in the bottle changed with time. These relationships would allow me to predict the outflow rate at any time, as well as how much water was left in the container at any time.

Data collection:

To measure height versus time, we marked the side of the bottle (within the cylindrical region) in one centimeter increments and recorded the time it took for the water level to drop from one mark to the next. There were a total of 11 marks.
To measure the outflow rate, we intercepted the outflow using a 25 ml beaker (not shown in Figure 2), and measured the time it took to fill to the 25 ml mark.

Results

Time elapsed since last measurement	Height of water in container	Time to fill 25ml beaker
Δt (s)	h (cm)	t_f (s)
0.0	11	6.4
45.5	10	7.1
52.3	9	8.5
43.1	8	7.1
56.1	7	7.8
60.6	6	8.3
57.6	5	8.9
65.0	4	9.9
72.8	3	11.2
76.7	2	13.0
91.1	1	14.8
122.0	0	17.3

Table 1: Outflow rate, water height change with time.

To analyze the data, we calculated the total time (the cumulative sum of the elapsed time since the previous measurement) and the outflow rate. The outflow rate is the change in volume with time:

$\text{outflow rate} = \frac{volume}{time} = \frac{dV}{dt} = \frac{25 ml}{t_f}$

So our data table becomes:

Time	Height of water in container	Outflow rate
t (s)	h (cm)	dV/dt (ml/s)
0.0	11	3.91
45.5	10	3.52
97.8	9	2.94
140.9	8	3.52
197	7	3.21
257	6	3.01
315.1	5	2.81
380.1	4	2.53
452.9	3	2.23
529.6	2	1.92
620.7	1	1.69
742.7	0	1.45

Table 2. Height of water and outflow rate of the bottle.

The graph of the height of the water with time shows a curve, although it is difficult to determine precisely what type of curve. My students started by trying to fit a quadratic equation to it, which should work as we’ll see in a minute.

h-v-t — Figure 3. The decrease in height with time is not a straight line (is non-linear).

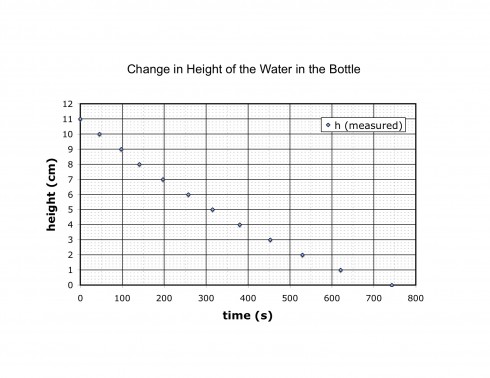
The plot of the outflow rate versus time, however, shows a pretty good linear trend. (Note that we do not use the first three datapoints (in Table 2), which we believe are erroneous because we were still sorting out the measuring method.)

Although I’ll note here that the data should ideally be modeled using a square root equation (Torricelli’s Law), that is beyond the present scope of the problem (we’ll try that tomorrow as a follow exercise).

Plotting the data in Excel we could add a linear trendline.

q-v-t — Figure 4. The outflow rate decreases linearly with time.

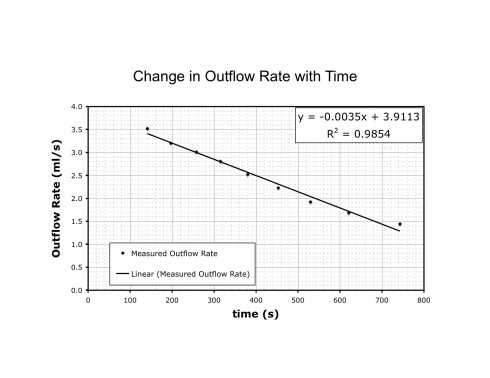
For the linear trendline, Excel gives the equation:

$y = -0.0035 x + 3.9113$

The y-axis is outflow rate (the change in volume with time), and the x axis is time (t), so the linear equation becomes:

$\frac{dV}{dt} = -0.0035 t + 3.9113$

Notice that this is a differential equation.

To determine the rate of at which the height of water in the container is changing, we need to recognize that the container is cylindrical in shape, and the volume (V) of a cylinder depends on its radius (r) and height (h):

$V = \pi r^2 h$

which can be substituted into differential in the rate equation:

$\frac{d(\pi r^2 h)}{dt} = -0.0035 t + 3.9113$

since π and the radius (r) are constants (since the jug’s shape is a cylinder), they can be pulled out of the differential:

$\pi r^2 \frac{dh}{dt} = -0.0035 t + 3.9113$

Dividing through by πr² solves for the rate of change of height:

$\frac{dh}{dt} = \frac{-0.0035 t + 3.9113}{\pi r^2}$

Isolating the coefficients gives:

$\frac{dh}{dt} = \frac{-0.0035}{\pi r^2} t + \frac{3.9113}{\pi r^2}$

This equation should give the rate at which the height changes with time, however, if you look at it carefully you’ll realize that for the time range we’re using (less than 800 seconds) the value of dh/dt will always be positive. We correct this by recognizing that the outflow rate is a loss of water, so a positive outflow should result in a negative change in height, therefore we rewrite the equation as:

$-\frac{dh}{dt} = \frac{-0.0035}{\pi r^2} t + \frac{3.9113}{\pi r^2}$

which gives:

$\frac{dh}{dt} = \frac{0.0035}{\pi r^2} t - \frac{3.9113}{\pi r^2}$

Now comes the calculus

Now, we can use this rate equation to find the equation for height versus time by integrating with respect to time:

$\int \frac{dh}{dt} dt = \int \left( \frac{0.0035}{\pi r^2} t - \frac{3.9113}{\pi r^2} \right) dt$

to get:

$h = \frac{0.0035}{2 \pi r^2} t^2 - \frac{3.9113}{\pi r^2} t + c$

And all we have to do to the find the constant of integration is substitute in a known point, an initial value. As is often the case, the best point to use is the starting point where t=0 makes the rest of the calculations easier. In our case, when t=0, h=11:

$11 = \frac{0.0035}{2 \pi r^2} (0)^2 - \frac{3.9113}{\pi r^2} (0) + c$

so:

$c = 11$

And our final equation becomes:

$h = \frac{0.0035}{2 \pi r^2} t^2 - \frac{3.9113}{\pi r^2} t + 11$

which is a quadratic equation as my students guessed before we did the calculus.

Does it work?

You will notice that in the math above, we never use the height data in determining equation for height versus time; all the calculations are based on the trendline for the outflow rate versus time.

As a result, we can compare the results of our equation to the actual measurements to see if our calculations are even close. Remarkably, they are.

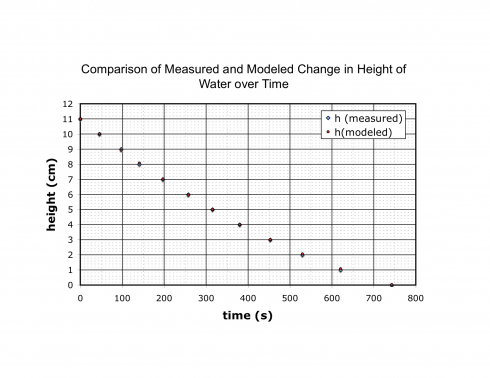
h-v-t-model-v-measured — Figure 5. The results from our equation (modeled) match the measured heights so well, the data points are difficult to distinguish on the graph.

Discussion

Despite all the potential for error, particularly, our relatively crude measurement techniques, and the imperfect cylindrical shape of our plastic bottle, the experiment went remarkably well.

Students found it quite challenging, and required some assistance even though this is a problem they have seen before in their textbook.

The problems in the textbook use Torricelli’s Law, which should much better describe the draining of a tank than the linear equation we find for dV/dt.

Torricelli’s Law:

$\frac{dV}{dt} = a \sqrt{2gh}$

where a is the area of the outlet hole, and g is the acceleration due to gravity.

In our actual experiment it is difficult to tell that a square root function would work better. Excel does not have an option for matching a square root function, so the calculations would become more involved (although it could be set up using Excel’s iterative solver or Goal Seek function).

Conclusion

Our experiment to use calculus to determine the rate of change of the height of water in a leaking plastic water bottle was a successful exercise even though the roughness of the data collection did not permit identification of the square root law for leakage.

Ultra-Violet Vision: Seeing like the Butterflies and the Bees

vis-uv-flower — Visible light (what we see) versus including ultra-violet light (what the bees see). Images by Klaus Schmitt: http://www.pbase.com/kds315/uv_photos

Dr. Klaus Schmitt has some utterly amazing photographs that simulate what bees and butterflies can see. They can see ultra-violet wavelengths of light, which we can’t.

Schmitt maps the ultra-violet in the image to blue to make it visible to our eyes.

His site (Photography of the Invisible World (updated)) has a lot more pictures and information about the process.

Monet’s Ultra-violet Vision

monet-uv-vision — Monet's two versions of "The House Seen from the Rose Garden" show the same scene as seen through his left (normal) and right eyes.

The eye’s lens is pretty good at blocking ultra-violet light, so when Claude Monet (whose works we visited earlier this year) had the lens of his eye removed he could see a little into the ultra-violet wavelengths of light.

Monet’s story is in a free iPad book put out by the Exploratorium of San Francisco called Color Uncovered (which I have to get). Carl Zimmer has a review that includes more details about Monet and how the eye works.

↬ Joe Hanson

P.S.: All of Monet’s works can be found on WikiPaintings, a great resource for electronic copies of old paintings (that are out of copyright).

Painting the Universe: How Scientists Produce Color Images from the Hubble Space Telescope

galaxies-rgb-anim-300 — The images taken by the Hubble Space Telescope are in black and white, but each image only captures a certain wavelength (color) of light.

The Guardian has an excellent video that explains how the images from the Hubble Space Telescope are created.

Each image from most research telescopes only capture certain, specific colors (wavelengths of light). One camera might only capture red light, another blue, and another green. These are captured in black and white, with black indicating no light and white the full intensity of light at that wavelength. Since red, blue and green are the primary colors, they can be mixed to compose the spectacular images of stars, galaxies, and the universe that NASA puts out every day.

582125main_image_2045_946-710 — Three galaxies. This image is a computer composite that combines the different individual colors taken by the telescope's cameras. Image from the Hubble Space Telescope via NASA.

The process looks something like this:

galaxies-rgb-color-500 — How images are assembled. Note that the original images don't have to be red, blue and green. They're often other wavelengths of light, like ultra-violet and infra-red, that are not visible to the eye but are common in space. So the images that you see from NASA are not necessarily what these things would look like if you could see them with the naked eye.

NASA’s image of the day is always worth a look.

Generating (and Saving) Tones with SoX

I’ve been using the command line program SoX to generate tones for my physics demonstrations on sound waves.

Single frequency tones can be used for talking about frequency and wavelength, as well as discussing octaves.

Combine two tones allows you to talk about interference and beats.

SoX can do a lot more than this, so I though I’d compile what I’m using it for in a single, reference post. For the record: I’m using SoX in Terminal on a Mac.

Using SoX

To play a single note (frequency 173.5 Hz) for 5 seconds, use:

> play -n  synth 5 sin 347

To save the note to a mp3 file (called note.mp3) use:

> sox -n note.mp3 synth 5 sin 347

The SoX command to play two notes with frequencies of 347 and 357 Hz is:

> play -n synth 15 sin 347 sin 357

and to make an mp3 file use:

> sox -n beat_10.mp3 synth 15 sin 347 sin 357

Listen for the Beat

beats — Two sound waves with slightly different frequencies sometimes cancel each other out (destructive interference) and sometimes add together (constructive interference) to create a sound that gets loud and quiter with a beat. The two lower sound waves (green and blue) are out of phase, and their combination (superposition) creates the third (red) wave.

Play two sound tones that are close together in frequency and the sound waves will overlap to create a kind of oscillating sound called a beat.

beats-2-anno — When you hear the beat (see below), you're hearing the alternating of the high amplitude region and the low amplitude region.

Below are two tones: separated and then mixed — listen for the beat.

	Frequency	Sound File (mp3)
Tone 1	347 Hz	1m.mpg
Tone 2	357 Hz	1m-357.mp3
Mixed Tones (with beat)	347 Hz + 357 Hz	beat_10.mp3

Interestingly, you can sometimes hear the beat as a third tone if the frequency difference is just right. The frequency of the beat is the difference between the frequency of the two tones.

Notes

The SoX command to play two notes with frequencies of 347 and 357 Hz is:

> play -n synth 15 sin 347 sin 357

to make an mp3 file use:

> sox -n beat_10.mp3 synth 15 sin 347 sin 357

Octave Sound Samples

I’ve not had much real musical training, but enough to know that I have a terrible ear for sound and can’t reproduce a note for anything. However, an informed source tells me that octaves represent the same note at different pitches.

The pitch is the frequency of the sound wave.

This "note" is a sound wave with a frequency (pitch) of 347 cycles per second (347 Hz), which has a wavelength of approximately 1 meter. It sounds like this.

If one note has twice the frequency of the other, they’re said to be one octave apart. For example, click on the image below to listen to the same note at different octaves:

Harmonic_octaves — Click the waves to hear the different octaves. The wavelengths of the sounds are shown (in meters).

Or play the files:

Wavelength	Frequency	Sound File (mp3)
1 m	347 Hz	1m.mpg
0.5 m	694 Hz	50cm.mp3
0.25 m	1388 Hz	25cm.mp3

How Black? 99.7% Black

One of my students asked, “How black can you get?” I didn’t know the answer; however, serendipitously, I ran into this article last night. Researchers in Rochester, NY have created a solar cell that absorbs 99.7% of incoming light, which means that it has an albedo (reflectivity) of just 0.3%. Since solar cells create energy by absorbing light, the more light it can absorb — the blacker the solar cell — the more efficient the solar cell is likely to be.