Tag: radioactivity

Linearizing an Exponential Function: Radioactive Decay

Using this data for the decay of a radioisotope, find its half life.

t (s)A (g)
0100
10056.65706876
20032.10023441
30018.18705188
40010.30425049
5005.838086287
6003.307688562

We can start with the equation for decay based on the half life:

   A = A_0 (\frac{1}{2})^\frac{t}{\lambda}  

 
where:
 A = \text{Amount of radioisotope (usually a mass)}  A_0 = \text{Initial amount of radioisotope (usually a mass)}   t = \text{time}  \lambda = \text{half life}

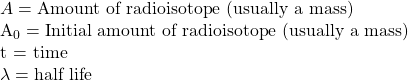
and linearize (make it so it can be plotted as a straight line) by using logarithms. 

Take the log of each side (use base 2 because of the half life):

  \log_2{(A)} = \log_2{  \left( A_0 (\frac{1}{2})^\frac{t}{\lambda} \right)} 

Use the rules of logarithms to simplify:

 \log_2{(A)} = \log_2{ ( A_0 )} + \log_2{  \left( (\frac{1}{2})^\frac{t}{\lambda} \right)}   

  \log_2{(A)} = \log_2{ ( A_0 )} +  \frac{t}{\lambda}  \log_2{   (\frac{1}{2}) }      

 \log_2{(A)} = \log_2{ ( A_0 )} +  \frac{t}{\lambda}  (-1)   

  \log_2{(A)} = \log_2{ ( A_0 )} -  \frac{t}{\lambda}       

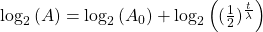
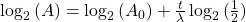
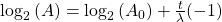
Finally rearrange a little:

  \log_2{(A)} =  -  \frac{t}{\lambda}  +  \log_2{ ( A_0 )}      

  \log_2{(A)} =  -  \frac{1}{\lambda} t +  \log_2{ ( A_0 )}

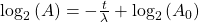
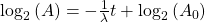
Now, since the two variables in the last equation are A and t we can see the analogy between this equation and the equation of a straight line:

 \log_2{(A)} =  -  \frac{1}{\lambda} t +  \log_2{ ( A_0 )}        

and,

   y =  m x +  b       

where:

   y = \log_2{(A)}        

   m =  -  \frac{1}{\lambda}        

   x = t       

   b =  \log_2{ ( A_0 )}

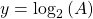
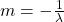
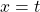
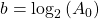
So if we draw a graph with log₂(A) on the y-axis, and time (t) on the x axis, the slope of the line should be:

   m =  -  \frac{1}{\lambda}

Which we can use to find the half life (λ).

Radioactive Half Lives

Since we most commonly talk about radioactive decay in terms of half lives, we can write the equation for the amount of a radioisotope (A) as a function of time (t) as:

  A = A_0 (\frac{1}{2})^\frac{t}{\lambda} 

where:
 A = \text{Amount of radioisotope (usually a mass)}  A_0 = \text{Initial amount of radioisotope (usually a mass)}   t = \text{time}  \lambda = \text{half life}

To reverse this equation, to find the age of a sample (time) we would have to solve for t:

Take the log of each side (use base 2 because of the half life):

  \log_2{(A)} = \log_2{  \left( A_0 (\frac{1}{2})^\frac{t}{\lambda} \right)} 

Use the rules of logarithms to simplify:

 \log_2{(A)} = \log_2{ ( A_0 )} + \log_2{  \left( (\frac{1}{2})^\frac{t}{\lambda} \right)}   

  \log_2{(A)} = \log_2{ ( A_0 )} +  \frac{t}{\lambda}  \log_2{   (\frac{1}{2}) }      

 \log_2{(A)} = \log_2{ ( A_0 )} +  \frac{t}{\lambda}  (-1)   

 \log_2{(A)} = \log_2{ ( A_0 )} -  \frac{t}{\lambda}      

Now rearrange and solve for t:

 \log_2{(A)} - \log_2{ ( A_0 )} = -  \frac{t}{\lambda}      

 -\lambda \left( \log_2{(A)} - \log_2{ ( A_0 )} \right) = t      

  -\lambda \cdot \log_2{ \left( \frac{A}{A_0} \right)}  = t

So we end up with the equation for time (t):

  t = -\lambda \cdot \log_2{ \left( \frac{A}{A_0} \right)}

Now, because this last equation is a linear equation, if we’re careful, we can use it to determine the half life of a radioisotope. As an assignment, find the half life for the decay of the radioisotope given below.

t (s)A (g)
0100
10056.65706876
20032.10023441
30018.18705188
40010.30425049
5005.838086287
6003.307688562

What Happens When Two Black Holes Collide?

A student asked this question about black holes during a discussion, and I didn’t have a good answer. Now there’s this:

A study last year found unusually high levels of the isotope carbon-14 in ancient rings of Japanese cedar trees and a corresponding spike in beryllium-10 in Antarctic ice.

The readings were traced back to a point in AD 774 or 775, suggesting that during that period the Earth was hit by an intense burst of radiation, but researchers were initially unable to determine its cause.

Now a separate team of astronomers have suggested it could have been due to the collision of two compact stellar remnants such as black holes, neutron stars or white dwarfs.

— via The Weather Channel (2013): Black Hole Collision May Have Irradiated Earth in 8th Century.

From the original article:

While long [Gamma Ray Bursts (GRBs)] are caused by the core collapse of a very massive star, short GRBs are explained by the merger of two compact objects … [such as] a neutron star with either a black hole becoming a more massive black hole, or with another neutron star becoming either a relatively massive stable neutron star or otherwise a black hole.

— Hambaryan and Neuhäuser (2013): A Galactic short gamma-ray burst as cause for the 14C peak in AD 774/5 in

More info via The Telegraph, and the original article discussing the spike in carbon-14 in tree rings is here.

Curve Matching: Radioactive Decay and the Distance Between the Earth and the Sun

According to theory, radioactive elements will always at a constant rate, with a little variability due to randomness. What you should not expect to find is that the rate of decay changes with the distance of the Earth from the Sun.

The rate of radioactive decay of Chlorine-36 (blue x's) seems to be related to the distance between the Earth and the Sun (red line). (Image from Dekant, 2012).

In pre-Calculus, we’re figuring out how to match curves to data. The scientists in this study do something similar, trying to see what types of sinusoidal curves will match the data, then seeing what natural phenomena have the same period (the time it takes for one cycle).

A Fatal Dose of Bananas

Banana: 1 μS.

In my last physics exam, I asked how many bananas would it take to deliver a fatal dose of radiation. This question came up when we were discussing different types of radiation and looking at this graph. One banana gives you about 0.1 microSieverts, while the usually fatal dosage is about 4 Sieverts. That means 4 million bananas. Michael Blastland uses the instantly fatal dosage of 8 Sieverts to make his estimate of eight million.

Usually Fatal Dose: 4 S.

My students were insistent, “Would eating four million bananas really kill you with radiation?”

My answer was, “Yes. But other problems might arise if you try to eat four million bananas.”